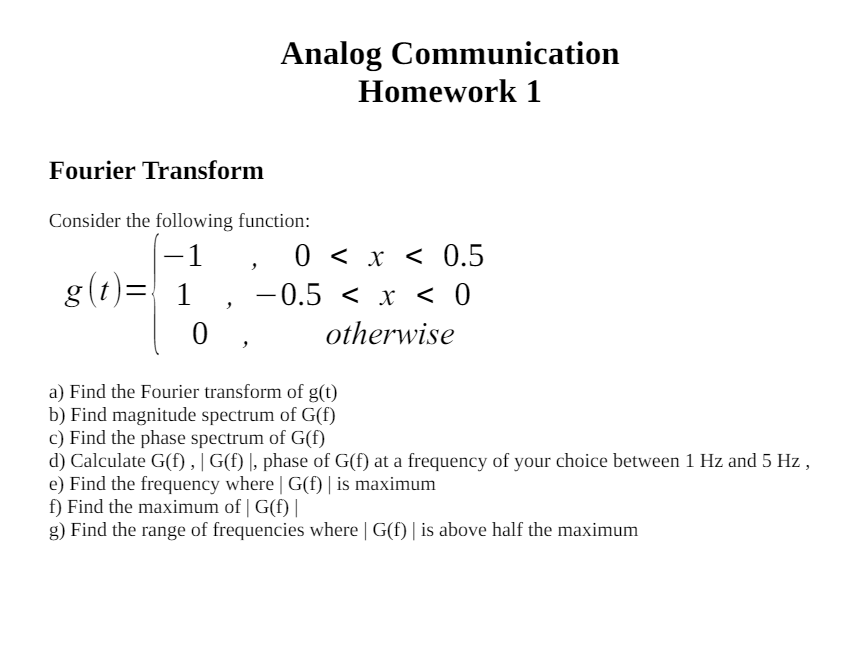
Analog Communication Homework 1 Fourier Transform Consider the following function: g(t) = { −1, 0 < x < 0.5 1, −0.5 < x < 0 0, otherwise a) Find the Fourier transform of g(t) b) Find magnitude spectrum of G(f) c) Find the phase spectrum of G(f) d) Calculate G(f), |G(f)|, phase of G(f) at a frequency of your choice between 1 Hz and 5 Hz, e) Find the frequency where |G(f)| is maximum f) Find the maximum of |G(f)| g) Find the range of frequencies where |G(f)| is above half the maximum