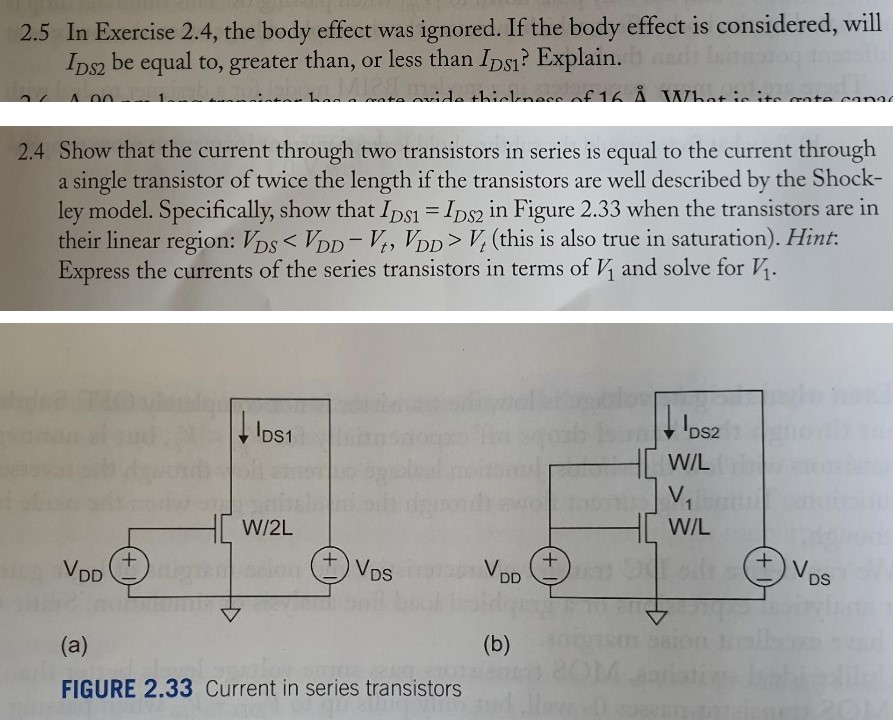
Ignore the body effect, show that the current through two transistors in series is equal to the current through a single transistor of twice the length if the transistors are well described by the Shockley 1st -order transistor model. Specifically, show that IDS1 = IDS2 in the following figures when the transistors are in their linear region: VDS < VDD - Vt, VDD > Vt. Hint: Express the currents of the series transistors in terms of V1 and solve for V1. If the body effect is now considered, will IDS2 be equal to, greater than, or less than IDS1? Please explain the reason.


You'll get a detailed, step-by-step and expert verified solution.
 Work With Experts to Reach at Correct Answers
Work With Experts to Reach at Correct Answers