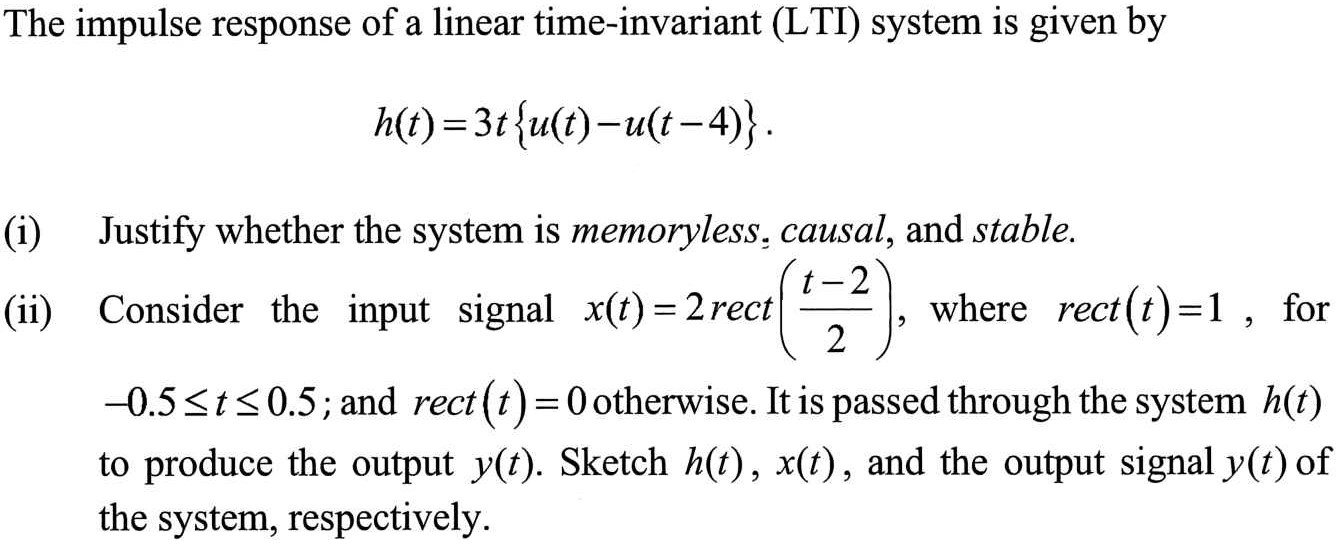
The impulse response of a linear time-invariant (LTI) system is given by h(t) = 3 t{u(t) − u(t−4)}. (i) Justify whether the system is memoryless, causal, and stable. (ii) Consider the input signal x(t) = 2 rect(t−2 2), where rect(t) = 1, for −0.5 ≤ t ≤ 0.5; and rect(t) = 0 otherwise. It is passed through the system h(t) to produce the output y(t). Sketch h(t), x(t), and the output signal y(t) of the system, respectively.
![Consider a linear, time-invariant system that has an impulse response of: {h[n]} = 12{δ[n−1]} + 12{δ[n+1]} a. Is the system causal? b. Is the system Bounded-input/Bounded-output stable? c. If the input signal is given by {f[n]} = 2{δ[n]} + {δ[n−1]} − {δ[n−2]} calculate and sketch the resulting output signal. d. What are the numerical values of the frequency response of the system at ω = 0 and ω = π?](https://www.doubtrix.com/uploads/editor/4610129288CXEMbLfuIB.png)
