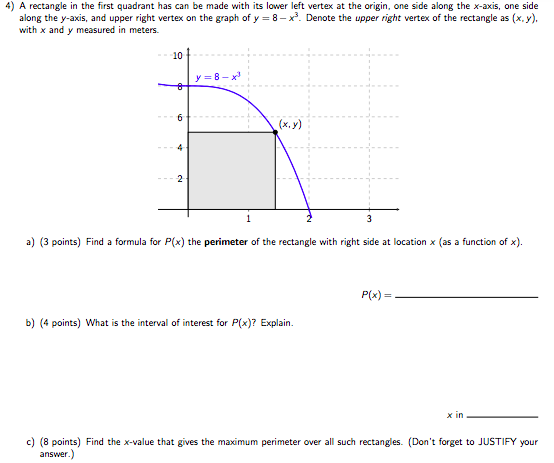
A rectangle in the first quadrant has can be made with its lower left vertex at the origin, one side along the x-axis, one side along the y-axis, and upper right vertex on the graph of y = 8−x3. Denote the upper right vertex of the rectangle as (x, y), with x and y measured in meters. a) (3 points) Find a formula for P(x) the perimeter of the rectangle with right side at location x (as a function of x). P(x) = b) (4 points) What is the interval of interest for P(x)? Explain. x in c) (8 points) Find the x-value that gives the maximum perimeter over all such rectangles. (Don't forget to JUSTIFY your answer.)
![Definite integrals are useful to science because we can often describe natural phenomena using definite integrals. However, integrating functions arising in real-life science can be rather challenging. In this exercise, we see how having only the graph of some functions is enough to answer some questions in science. The physical interpretation of an integral is a prerequisite knowledge you learned in MATH 1013. For a refresher, see the Net Change Theorem in Section 5.4 of the textbook. A group of researchers studies the population of arctic foxes in Northern Canada. Initially, the researchers identified a population of 1,000 arctic foxes. Due to various factors, the population of arctic foxes changes over time. The graph of the birthrate B(t) and the death rate D(t), for the population, are shown below. Time t is measured in years. (1) In a sentence or two, describe the meaning of the function f(t) = t 0 B(x) - D(x)dx in the context of this problem. (2) According the graph, is there a time t between 0 and 20 at which the population is equal to the initial population? If so, provide a rough estimate of this time, and write one or two sentences explaining how you found it. Your estimate should be a single number, not an interval. (3) Recall from Quiz 1 the definition of the average value of a function f(x). The average value of a function f(x) over an interval [a, b] is denoted by favg and is defined to be favg = 1 b - a b a f(x)dx. Suppose you know that the average birth rate over the first 20 years is 90 arctic foxes per year and the average death rate over the first 20 years is 100 arctic foxes per year. What is the population of the arctic foxes at t = 20 ? Show your work.](https://www.doubtrix.com/uploads/editor/1288831183fhExDQGbmw.png)
