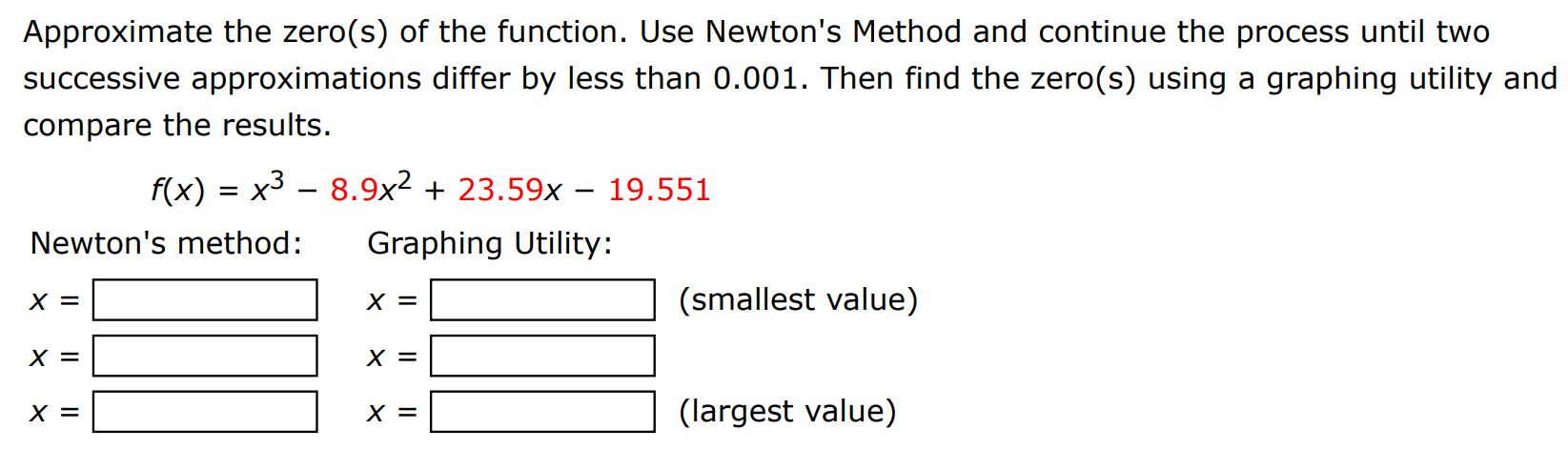
Approximate the zero(s) of the function. Use Newton's Method and continue the process until two successive approximations differ by less than 0.001 . Then find the zero(s) using a graphing utility and compare the results. f(x) = x3 − 8.9x2 + 23.59x − 19.551 Newton's method: x = Graphing Utility: x = x = (smallest value) x = x = x = (largest value)