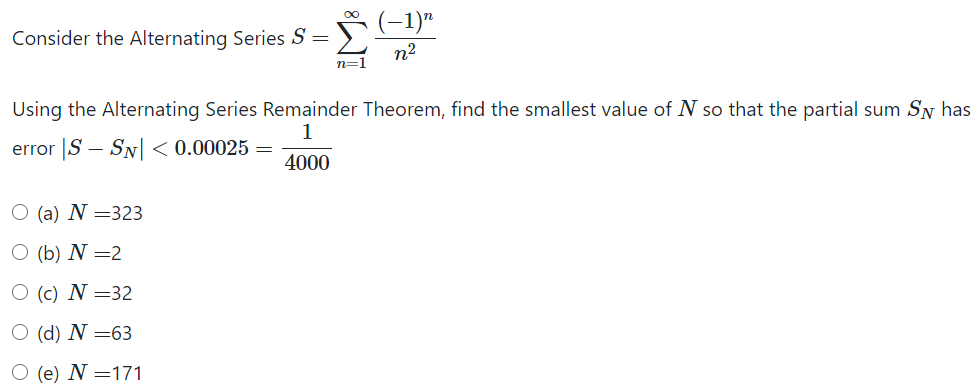
Consider the Alternating Series S = ∑ n = 1 ∞ (−1) n n 2 Using the Alternating Series Remainder Theorem, find the smallest value of N so that the partial sum SN has error |S − SN| < 0.00025 = 1/4000 (a) N = 323 (b) N = 2 (c) N = 32 (d) N = 63 (e) N = 171