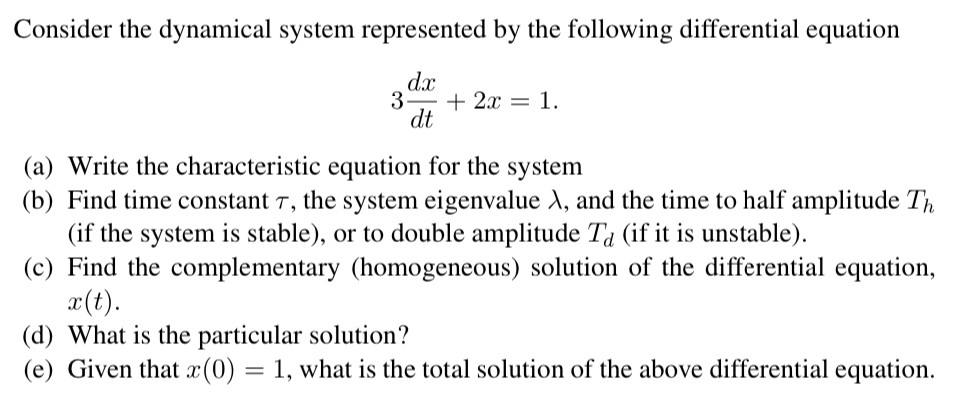
Consider the dynamical system represented by the following differential equation 3 dx dt + 2x = 1. (a) Write the characteristic equation for the system (b) Find time constant τ, the system eigenvalue λ, and the time to half amplitude Th (if the system is stable), or to double amplitude Td (if it is unstable). (c) Find the complementary (homogeneous) solution of the differential equation, x(t). (d) What is the particular solution? (e) Given that x(0) = 1, what is the total solution of the above differential equation.