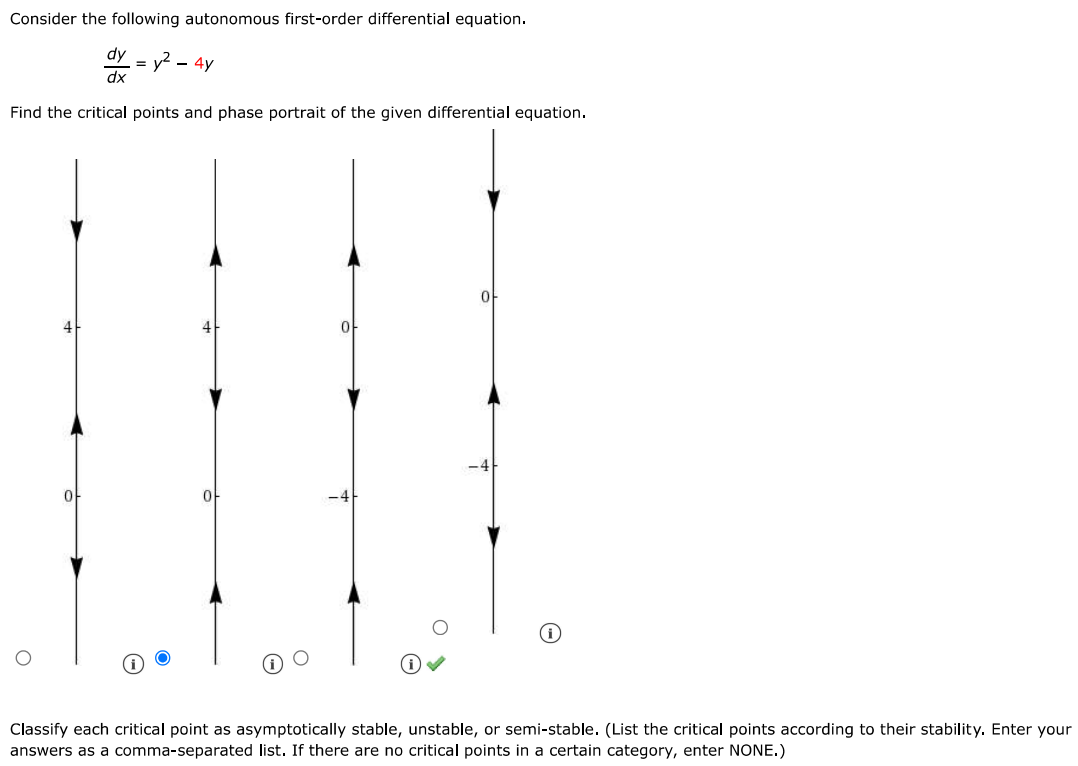
Consider the following autonomous first order differential equation. dy/dx=y^2-4y Find the critical points and the phase portrait of the given differential equation. Classify each critical point as asymptotically stable, unstable, or semi-stable (List the critical points according to their stability. Enter your answers as a comma-separated list. If there are no critical points in a certain category, enter NONE.) Sketch typical solution curves in the regions in the xy-plane determined by the graphs of the equilibrium solutions.


You'll get a detailed, step-by-step and expert verified solution.
 Work With Experts to Reach at Correct Answers
Work With Experts to Reach at Correct Answers