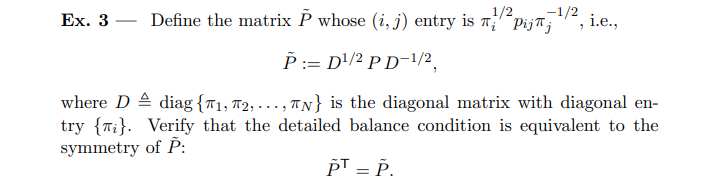
Ex. 3 - Define the matrix P˜ whose (i,j) entry is πi 1/2 pijπj -1/2 , i.e., P˜: = D 1/2PD -1/2 , where D ≜ diag{π1, π2, … , πN} is the diagonal matrix with diagonal entry {πi }. Verify that the detailed balance condition is equivalent to the symmetry of P˜ : P˜ ⊤ = P˜.
related to stochastic process dtmc model


You'll get a detailed, step-by-step and expert verified solution.
 Work With Experts to Reach at Correct Answers
Work With Experts to Reach at Correct Answers