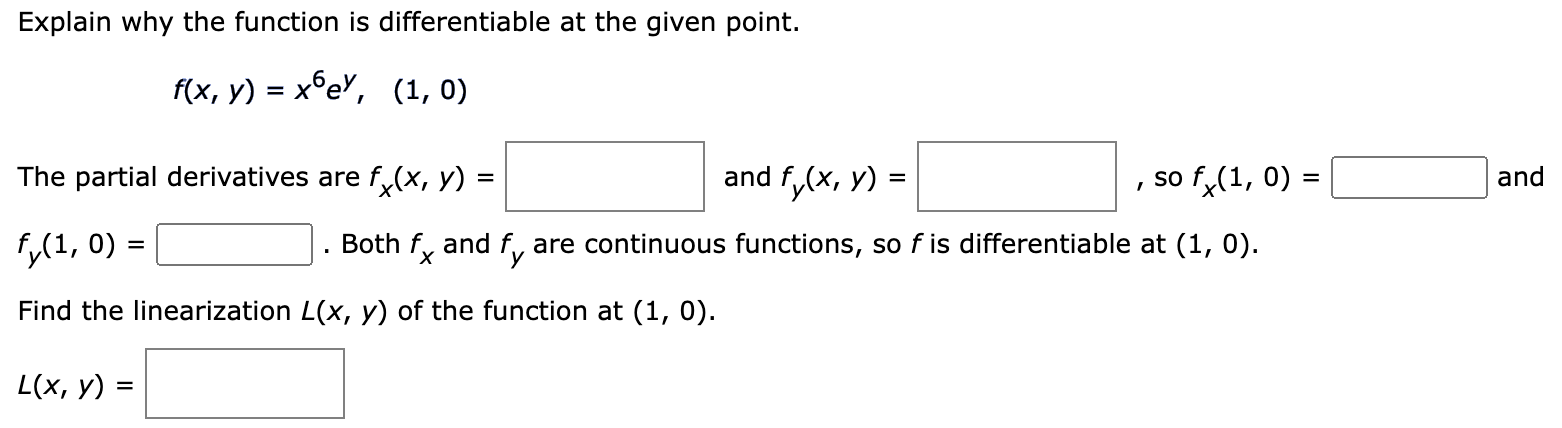
Explain why the function is differentiable at the given point. f(x, y) = x6ey, (1, 0) The partial derivatives are fx(x, y) = and fy(x, y) = , so fx(1, 0) = and fy(1, 0) = . Both fx and fy are continuous functions, so f is differentiable at (1, 0). Find the linearization L(x, y) of the function at (1, 0). L(x, y) =