Let R be the finite region bounded by curves y = 1/x and 3x + 3y = 10. A solid S is obtained by rotating the region R about the y-axis. Which of the following integral represents the volume of S ? Select one: a. π ∫ 3 1/3 1/x2 - 10-3x 3 2 dx b. π ∫ 3 1/3 1/y2 - 10-3y 3 2 dy c. π ∫ 3 1/3 10-3y 3 2 - 1/y2dy d. π ∫ 3 1/3 10-3x 3 2 - 1/x2 dx
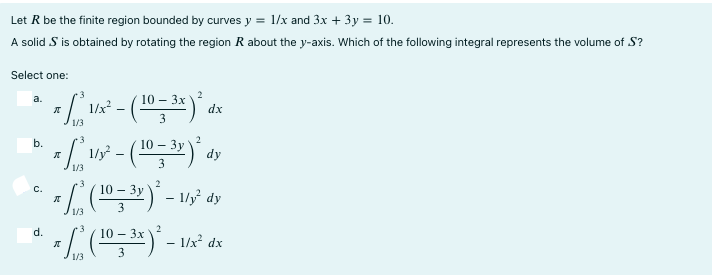
Explain how you got to the correct answer. THANK YOU
You'll get a detailed, step-by-step and expert verified solution.
Correct & Verified Answers
No AI-Generated Answers
Video Solution for Difficult Questions
Work With Experts to Reach at Correct Answers
![Define R as the region bounded by the graphs of f(x) = 3√x and g(x) = x^2 / 4 over the interval [1, 4]. Which of the following represents the volume of the solid of revolution formed by rotating R about the line x = -1 ∫1 4 2π(x + 1) (3√x – x^2 / 4 ) dx ○ ∫1 4 2π(x - 1) ( x^2 / 4 - 3√x) dx ∫1 4 2π(x - 1) (3√x – x^2 / 4 ) dx ∫1 4 2π(x + 1) ( x^2 / 4 - 3√x) dx ∫4 1 2π(x + 1) (3√x – x^2 / 4 ) dx](https://www.doubtrix.com/uploads/editor/5896888824LCQVfAnVrd.png)
