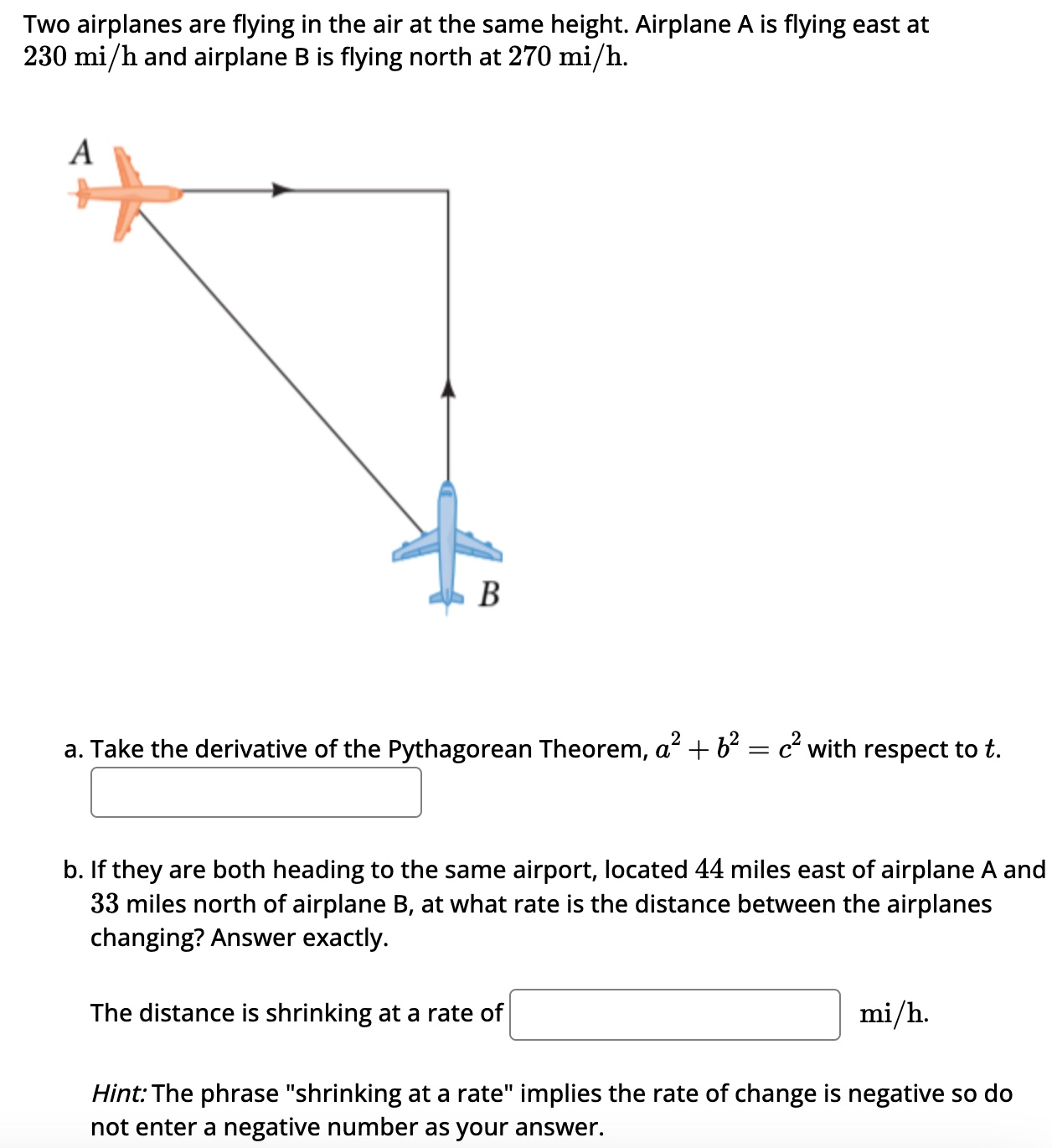
Two airplanes are flying in the air at the same height. Airplane A is flying east at 230 mi/h and airplane B is flying north at 270 mi/h. a. Take the derivative of the Pythagorean Theorem, a2 + b2 = c2 with respect to t. b. If they are both heading to the same airport, located 44 miles east of airplane A and 33 miles north of airplane B, at what rate is the distance between the airplanes changing? Answer exactly. The distance is shrinking at a rate of mi/h. Hint: The phrase "shrinking at a rate" implies the rate of change is negative so do not enter a negative number as your answer.