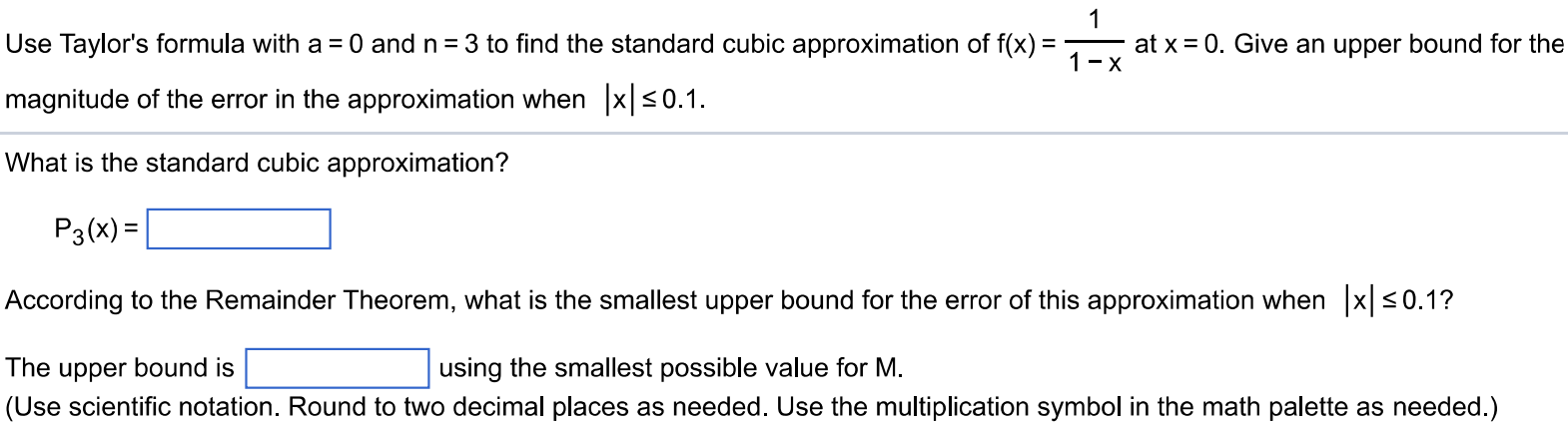
Use Taylor’s formula with a = 0 and n = 3 to find the standard cubic approximation of f(x) = 1/1-x at x = 0. Give an upper bound for the magnitude of the error in the approximation when |x| ≤ 0.1. What is the standard cubic approximation? P3(x) = According to the Remainder Theorem, what is the smallest upper bound for the error of this approximation when |x| ≤ 0.1 ? The upper bound is using the smallest possible value for M. (Use scientific notation. Round to two decimal places as needed. Use the multiplication symbol in the math palette as needed.)


You'll get a detailed, step-by-step and expert verified solution.
 Work With Experts to Reach at Correct Answers
Work With Experts to Reach at Correct Answers