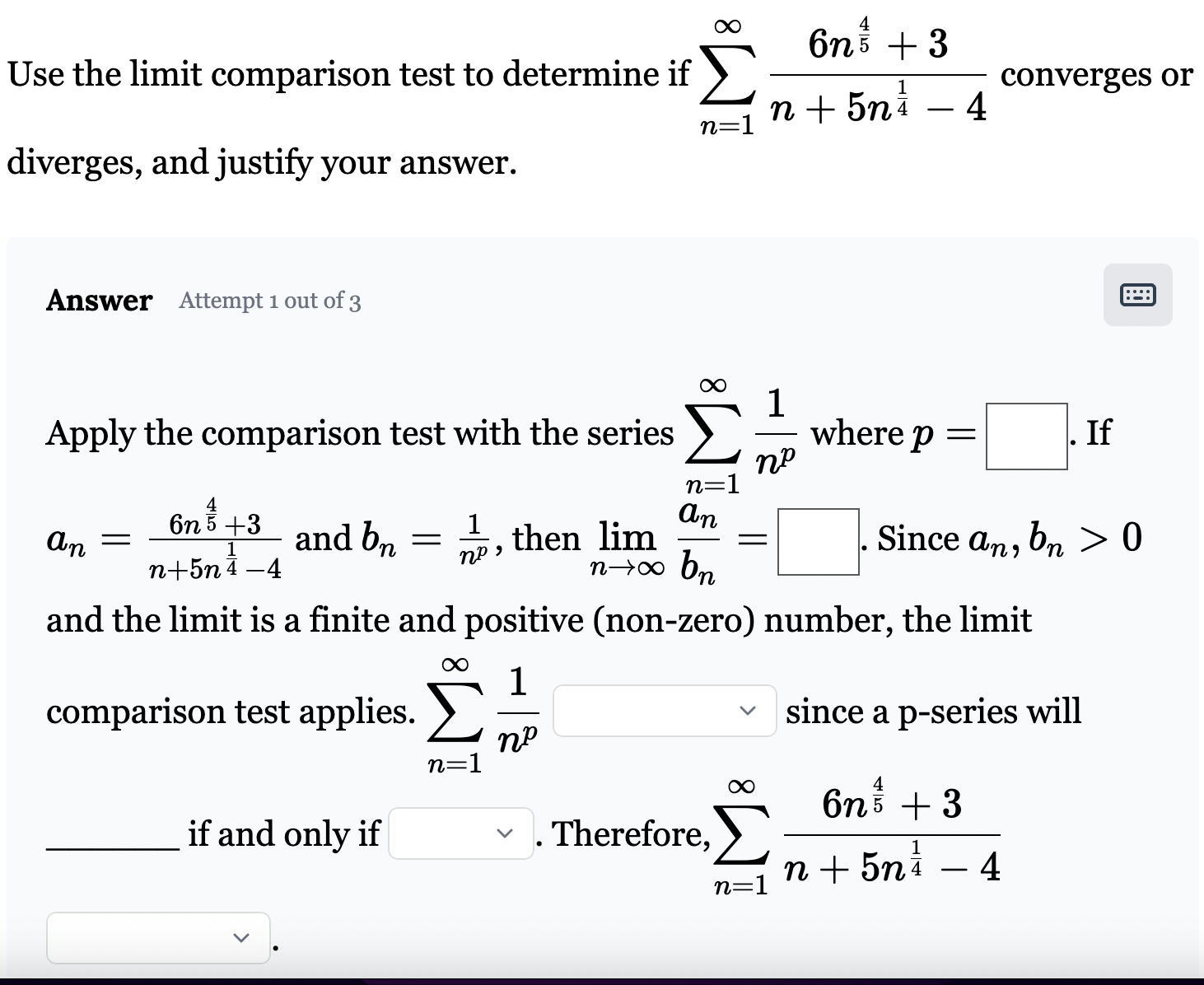
Use the limit comparison test to determine if ∑n = 1∞6 n45+3 n+5 n14−4 converges or diverges, and justify your answer. Answer Attempt 1 out of 3 Apply the comparison test with the series ∑n = 1∞1 np where p = . If an = 6 n45+3 n+5 n14−4 and bn = 1 np, then limn→∞an bn = . Since an, bn > 0 and the limit is a finite and positive (non-zero) number, the limit comparison test applies. ∑n = 1∞1 np since a p-series will if and only if . Therefore, ∑n = 1∞6 n45+3 n+5 n14−4