What is the minimum value of n that will guarantee the estimate of the

Question

Notify Me Bookmark

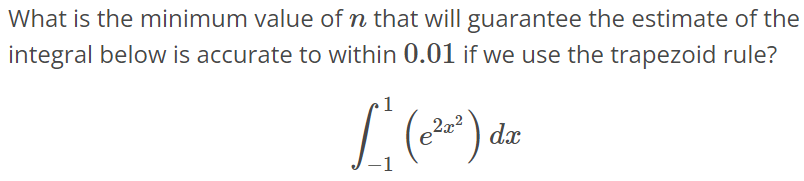
What is the minimum value of n that will guarantee the estimate of the integral below is accurate to within 0.01 if we use the trapezoid rule? ∫ 1 -1 (e 2x^2 )dx

What is the minimum value of n that will guarantee the estimate of the integral below is accurate to within 0.01 if we use the trapezoid rule? ∫ 1 -1 (e 2x^2 )dx

Image text

What is the minimum value of n that will guarantee the estimate of the integral below is accurate to within 0.01 if we use the trapezoid rule? ∫ 1 -1 (e 2x^2 )dx

Explanation & Steps

Use error bound formula for the trapezoidal rule to determine the minimum value of n, which provides maximum error within the given limits:

$Error\:in\:T_n \leq \frac{M(b-a)^3}{12n^2}$

Where, M is the maximum value of |f''(x)| over [a, b].

Detailed Answer

Answer

Ask Your Doubt Rate This Answer Request Video Solution

Previous question

Next question

Student Reviews:
(4)
Correct answers (3)
Complete solution (3)
Step-by-step solution (3)
Fully explained (3)

Submit Questions

Similar/Related Questions

Solution

Solution

Solution