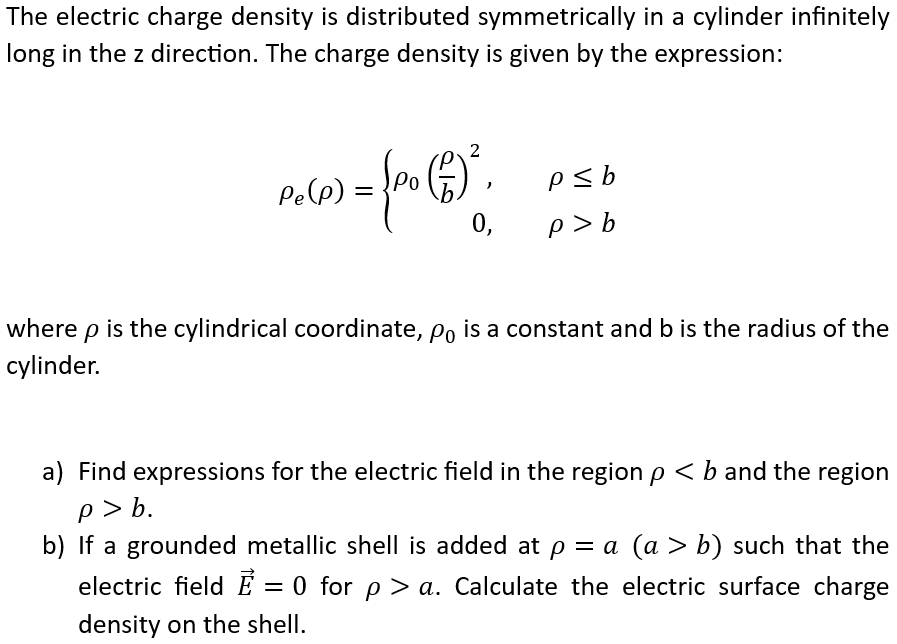
The electric charge density is distributed symmetrically in a cylinder infinitely long in the z direction. The charge density is given by the expression: ρe(ρ) = {ρ0(ρb)2, ρ ≤ b0, ρ > b where ρ is the cylindrical coordinate, ρ0 is a constant and b is the radius of the cylinder. a) Find expressions for the electric field in the region ρ < b and the region ρ > b. b) If a grounded metallic shell is added at ρ = a(a > b) such that the electric field E→ = 0 for ρ > a. Calculate the electric surface charge density on the shell.
![A total charge Q is uniformly distributed, with surface charge density σ, over a very thin disk of radius R. The electric field at a distance d along the disk axis is given by E→ = σ2ϵ0[1−dd2+R2]n^ where n^ is a normal unit vector perpendicular to the disk. What is the best approximation for the electric field magnitude E at large distances from the disk? a) σ2ϵ0 b) QRϵ0 d2 c) σR4ϵ0 d d) σϵ0(R2 d)2 e) none of the above](https://www.doubtrix.com/uploads/editor/0675279737hrIrQWHgba.png)