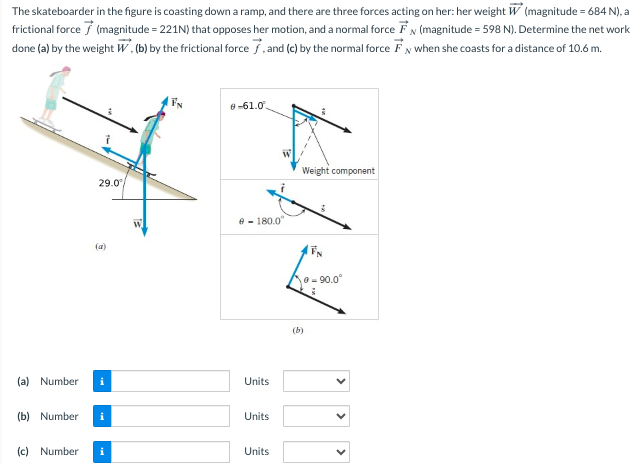
The skateboarder in the figure is coasting down a ramp, and there are three forces acting on her: her weight W→ (magnitude = 684 N), a frictional force f→ (magnitude = 221 N) that opposes her motion, and a normal force F→N (magnitude = 598 N). Determine the net work done (a) by the weight W→, (b) by the frictional force f→, and (c) by the normal force F→N when she coasts for a distance of 10.6 m. (a) (b) (a) Number Units (b) Number Units (c) Number Units